

Start your AP® exam prep today.\(\require
#Fundamental theorem of calculus part 1 how to#
Part 1 of the Fundamental Theorem tells us how to differentiate the Fresnel. Kickstart your AP® Calculus prep with Albert. Differential calculus arose from the tangent problem, whereas integral. Now, this theorem on its own is already useful, but it also supplies us with the fact that this definite integral is equivalent to the total change over a particular interval, which comes in handy in a number of situations as we saw in the last two problems above. The Fundamental Theorem of CalculusĪs you can see, the fundamental theorem of calculus establishes a procedure for calculating a definite integral. The process of finding the definite integral is called integration or integrating f(x). Confirm that the Fundamental Theorem of Calculus holds for several examples. When integrating this function, we are looking for a curve whose derivative is dt=8264 gallons. Definite IntegralsĪn indefinite integral is an integral without limits of integration for example, That is, you are integrating over an interval whose endpoints you use to evaluate the integral. Fundamental Theorem of Calculus Part 1 and 2: The fundamental theorem of calculus is a theorem in mathematics that states that the integral of a function. Recall that a definite integral is an integral where you are given the limits of integration. Part 1 of the Fundamental Theorem of Calculus (FTC) states that given F(x) x af(t)dt, F (x) f(x). The second part says that in order to find the definite integral of f f between a a and b b, find an antiderivative of f f, call it F F, and calculate F (b)-F (a) F (b) F (a).

antiderivative, Fundamental Theorem of Calculus (First and Second) Objectives. The first part says that if you define a function as the definite integral of another function f f, then the new function is an antiderivative of f f. The fundamental theorem of calculus states that differentiation and integration are inverse operations. This theorem establishes the procedure for computing a definite integral. Integrals: The Fundamental Theorem of Calculus Vocabulary. This implies the existence of antiderivatives for continuous functions. This video contain plenty of examples and practice problems.

Here, we will focus on the first statement, which is referred to as the First Fundamental Theorem of Calculus. The first part of the theorem, the first fundamental theorem of calculus, states that for a function f, an antiderivative or indefinite integral F may be obtained as the integral of f over an interval with a variable upper bound. 302K views 6 years ago This calculus video tutorial explains the concept of the fundamental theorem of calculus part 1 and part 2. The fundamental theorem of calculus states that if a function f(x) is continuous on the interval a, b, then there exists a function F(x), called the. There are two parts to the Fundamental Theorem: the first justifies the procedure for evaluating definite integrals, and the second establishes the relationship between differentiation and integration.
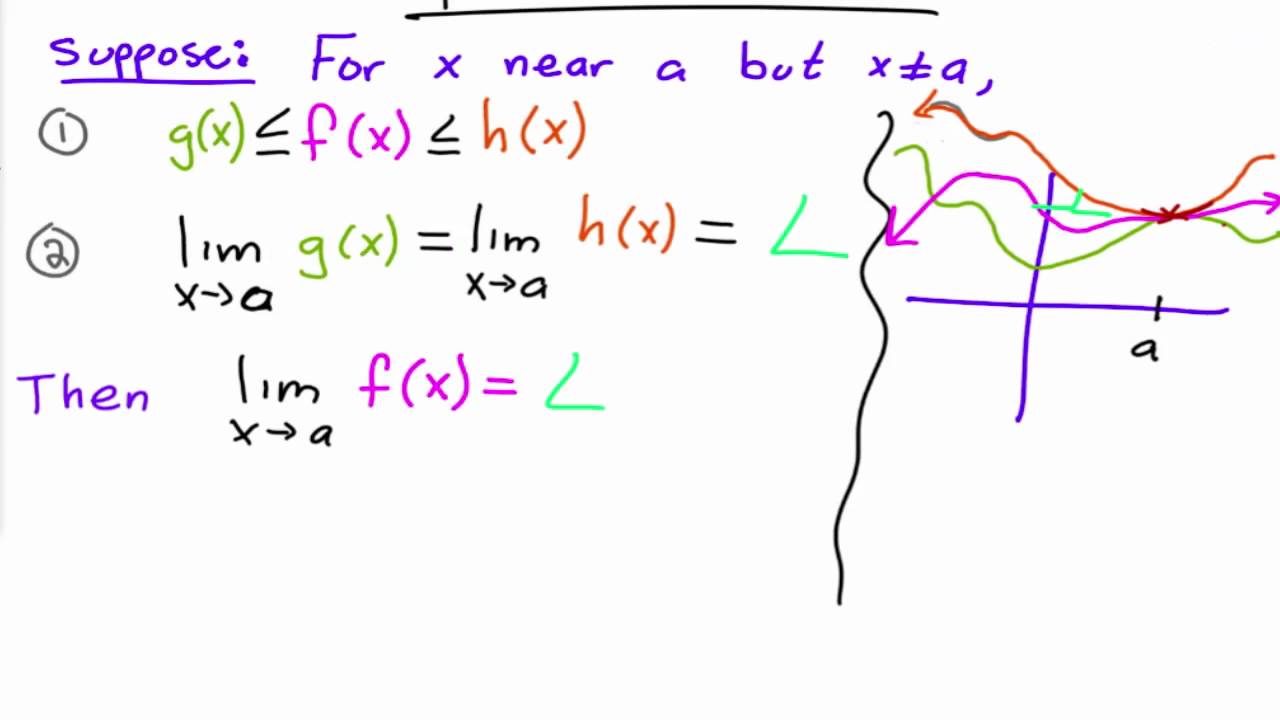
The Fundamental Theorem of Calculus brings together two essential concepts in calculus: differentiation and integration. That is, the derivative of a definite integral of f whose upper limit is the variable x and whose lower limit is the constant a equals the function f evaluated.


 0 kommentar(er)
0 kommentar(er)
